《Java 数据结构与算法》第8章：树(AVL)

小傅哥

发布于 2022-12-13 14:11:49

4920

发布于 2022-12-13 14:11:49

文章被收录于专栏：CodeGuide | 程序员编码指南

作者：小傅哥博客：https://bugstack.cn

❝沉淀、分享、成长，让自己和他人都能有所收获！😜 ❞

一、前言
二、AVL树数据结构
三、AVL树代码实现
- 1. 左旋
- 2. 右旋
- 3. 左旋 + 右旋
- 4. 右旋 + 左旋
四、AVL树功能测试
五、常见面试题

一、前言

AVL树历史

在计算机科学中，AVL 树以其两位苏联发明家Georgy Adelson-Velsky和 Evgenii Landis的名字命名，他们在 1962 年的论文“信息组织算法”中发表了它。它是一种自平衡二叉搜索树(BST)，这是发明的第一个这样的数据结构。

二、AVL树数据结构

AVL 自平衡二叉树的出现，其目的在于解决二叉搜索树退化成链表的问题。当我们向BST二叉搜索树顺序存入1、2、3、4、5、6、7个元素时，它会退化成一条链表，因而失去树查询的时间复杂度，所以我们需要AVL树平衡树高。如图所示

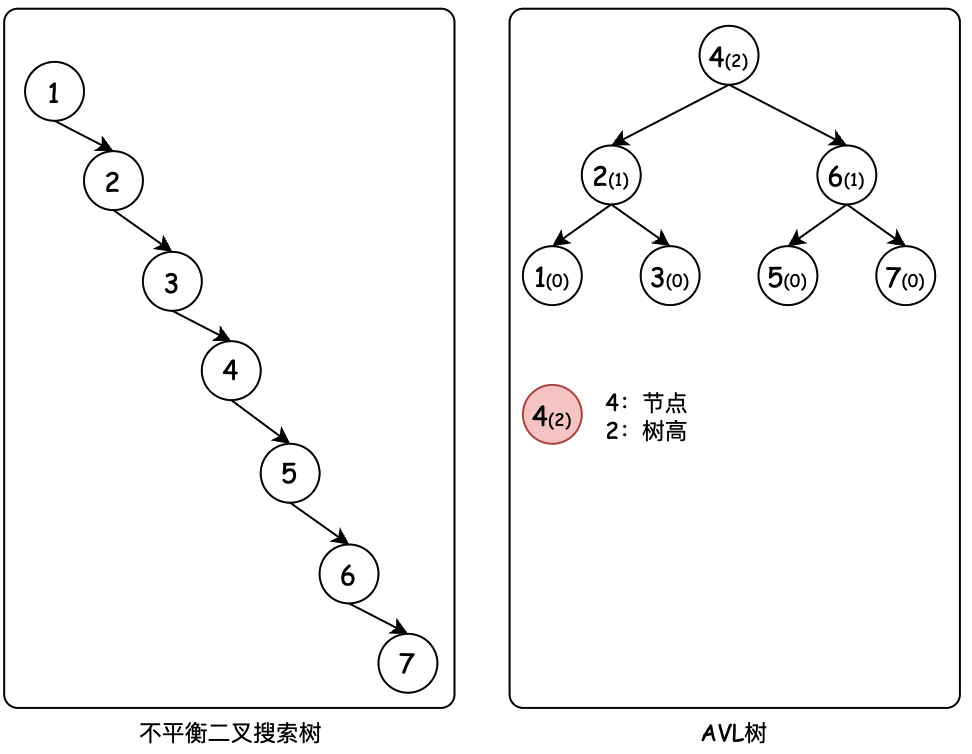
那么AVL树是怎么平衡树高的呢？

当二叉树的左右分支树高差不为1时，需要进行左旋或者右旋，来调衡树高。这有点像开车的时候，如果车头偏左就往右打方向盘，车头偏右就往左打方向盘是一个道理。那这个方向盘(左旋、右旋)是怎么打的呢，主要分以下四种情况；

节点树高：以节点4为说明，最长的左右分支节点个数，就是节点4的最大树高。这里节点4左右孩子节点最长路径都为2，所以它的树高为2。同理可计算其他节点树高。
平衡因子：通过当前节点的左右子节点作差计算平衡因子，之后AVL树通过平衡因子，定义了什么时候进行左旋和右旋。

三、AVL树代码实现

对于 AVL 树的实现与 BST 二叉搜索树相比，在树的节点定义上多了一个树高的属性。也有些AVL树使用的是平衡因子的属性，就是通过树高计算后的结果。树节点代码结构如下；

public class Node {

    public Class<?> clazz;
    public Integer value;
    public Node parent;
    public Node left;
    public Node right;
    // AVL 树所需属性
    public int height;
    
}

接下来小傅哥就分别通过代码讲解下一颗AVL树的左旋、右旋、左旋+右旋、右旋+左旋的代码操作。不要担心这没有多复杂，只要你能搞清楚左旋，就能搞清楚右旋。两旋弄懂组合就没啥难度了。

源码地址：https://github.com/fuzhengwei/java-algorithms
本章源码：https://github.com/fuzhengwei/java-algorithms/tree/main/data-structures/src/main/java/stack
动画演示：https://visualgo.net/zh/bst?slide=1 —— AVL树初次理解还是比较困难的，可以结合学习内容的同时做一些动画演示。

1. 左旋

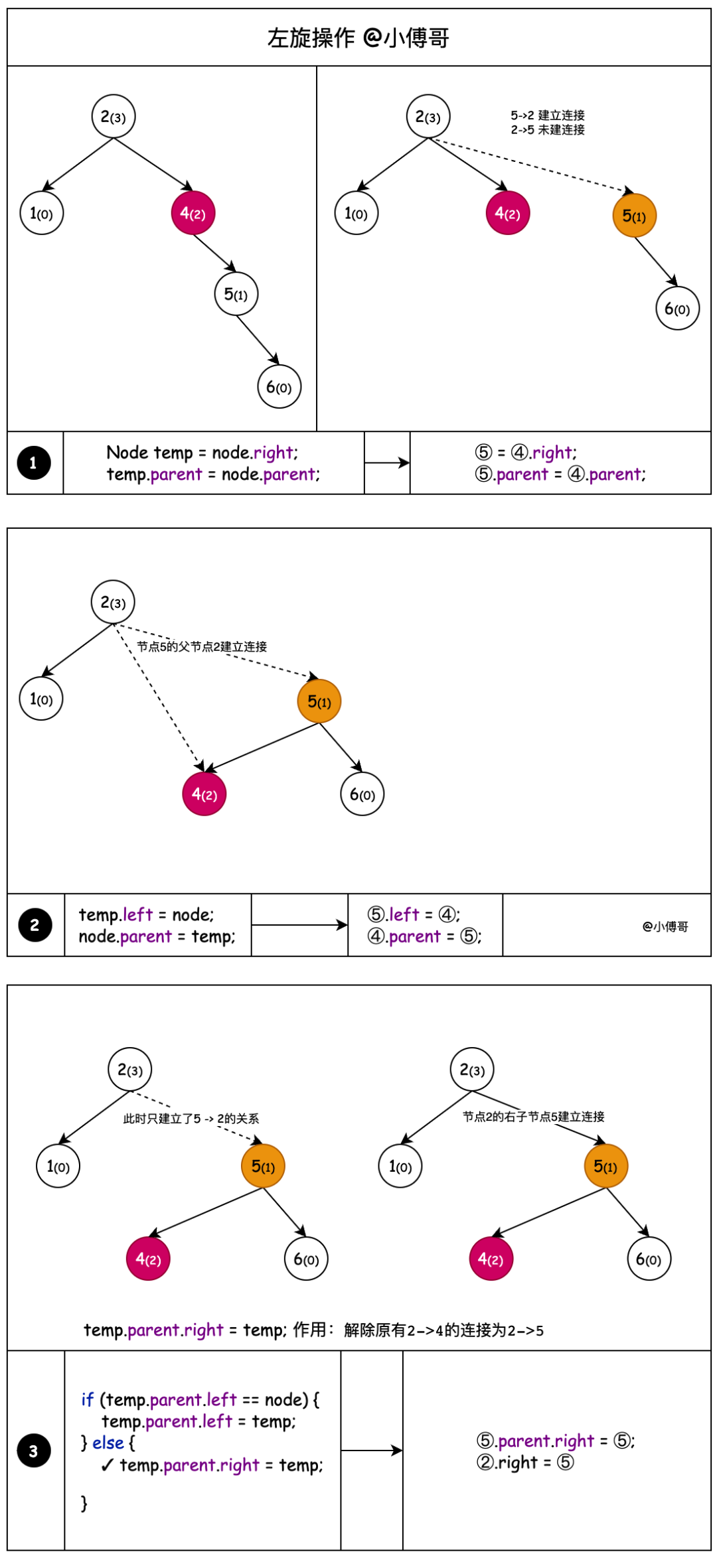
图解左旋操作；它就是一种摘链更换调整节点的处理过程，小傅哥把它分解展示，整个过程如下；

代码实现

protected Node rotateLeft(Node node) {
    Node temp = node.right;
    temp.parent = node.parent;
  
    node.right = temp.left;
    if (node.right != null) {
        node.right.parent = node;
    }
  
    temp.left = node;
    node.parent = temp;
  
    if (temp.parent == null) {
        root = temp;
    } else {
        if (temp.parent.left == node) {
            temp.parent.left = temp;
        } else {
            temp.parent.right = temp;
        }
    }
    return temp;
}

左旋的作用，相当于通过向上迁移树高差大于1的右子节点来降低树高的操作。
通过节点4拿到父节点2和右子节点5，把父节点2和右子节点5建立关联
节点5的左子节点，相当于是大于4小于4的那么一个值，只不过这里不体现。那么这个节点4的左子节点，应该被迁移到节点3的右子节点上。
整理节点5的关系，左子节点为4。左子节点4的父节点为5
如果说迁移上来的节点5无父节点，那么它就是父节点 root = temp
迁移上来的节点5，找到原节点4是对应父节点的左子节点还是右子节点，对应的设置节点5的左右位置

2. 右旋

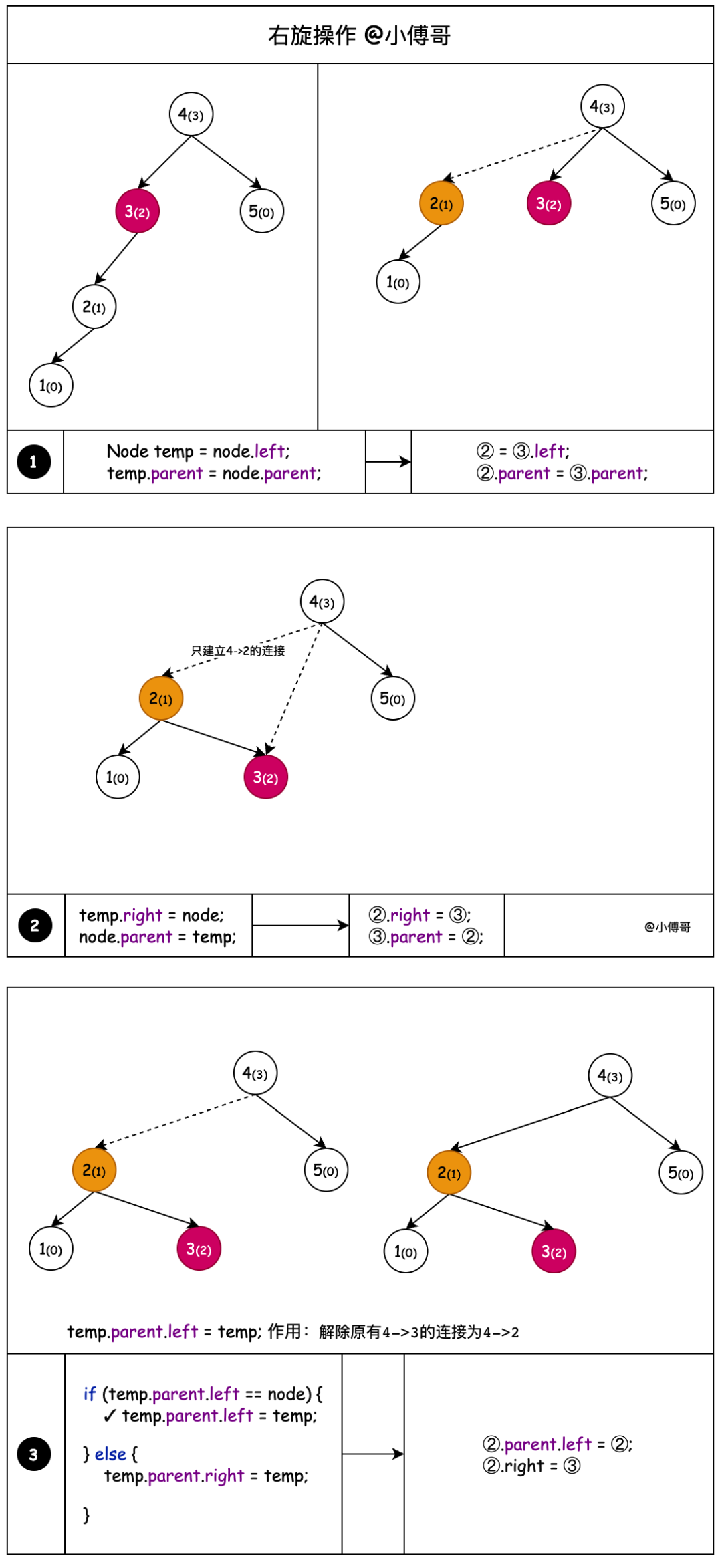
图解右旋操作；它就是一种摘链更换调整节点的处理过程，小傅哥把它分解展示，整个过程如下；

代码实现

protected Node rotateRight(Node node) {
    Node temp = node.left;
    temp.parent = node.parent;
    node.left = temp.right;
    if (node.left != null) {
        node.left.parent = node;
    }
    temp.right = node;
    node.parent = temp;
    if (temp.parent == null) {
        root = temp;
    } else {
        if (temp.parent.left == node) {
            temp.parent.left = temp;
        } else {
            temp.parent.right = temp;
        }
    }
    return temp;
}

右旋的作用，相当于通过向上迁移树高差大于1的右子节点来降低树高的操作。
通过节点3拿到父节点4和左子节点2，把父节点7和左子节点2建立关联
节点2的右子节点，相当于是大于2小于3的那么一个值，只不过这里不体现。那么这个节点2的右子节点，应该被迁移到节点3的左子节点上。
整理节点2的关系，右子节点为3。右子节点3的父节点为2
如果说迁移上来的节点2无父节点，那么它就是父节点 root = temp
迁移上来的节点2，找到原节点3是对应父节点的左子节点还是右子节点，对应的设置节点2的左右位置

3. 左旋 + 右旋

之所以会有左旋 + 右旋，是因为一次右旋操作没法平衡树高，而这种树的不平衡节点的左子节点的右子节点过长，所以要把不平衡节点的左子节点向左旋转一次，之后再进行右旋操作。

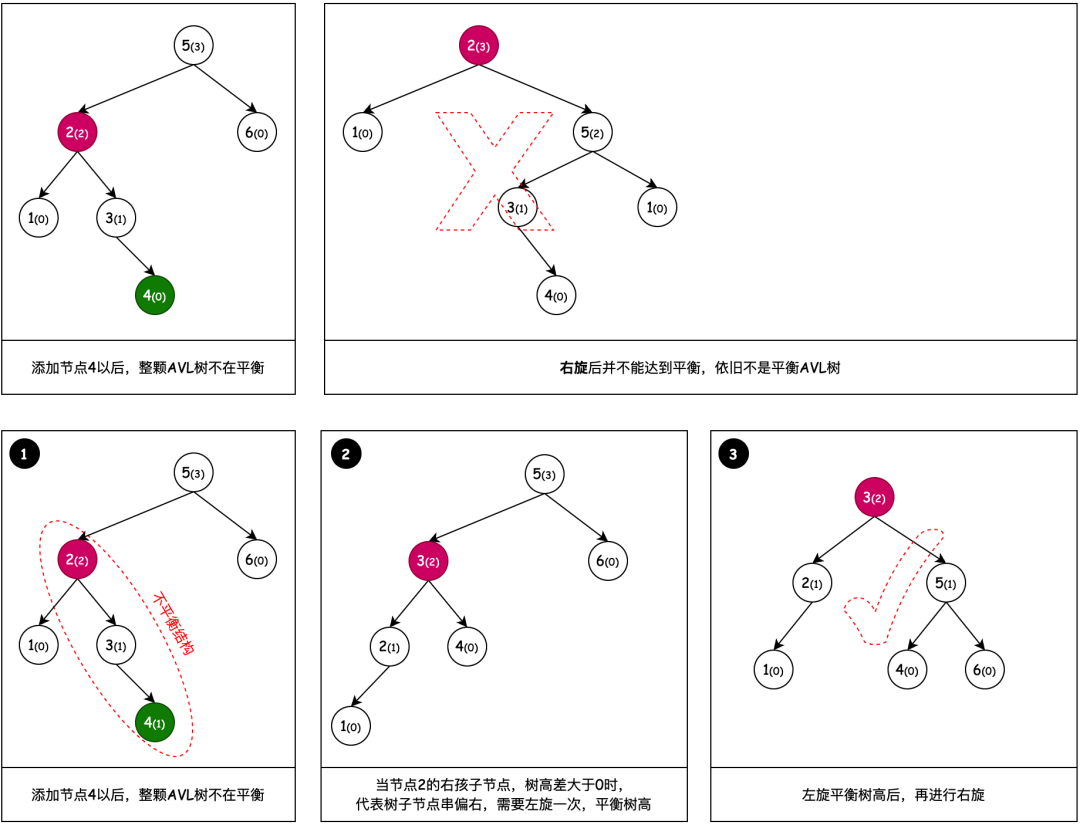
代码实现

if (factor(node.left) >= 0) {
    Node temp = super.rotateRight(node);
    refreshHeight(temp.right);
    refreshHeight(temp);
} else {
    Node temp = super.rotateLeft(node.left);
    refreshHeight(temp.left);
    refreshHeight(temp);
    node.left = temp;
    
    temp = super.rotateRight(node);
    refreshHeight(temp.right);
    refreshHeight(temp);
}

4. 右旋 + 左旋

之所以会有右旋 + 左旋，是因为一次左旋操作没法平衡树高，而这种树的不平衡节点的右子节点的左子节点过长，所以要把不平衡节点的右子节点向右旋转一次，之后再进行左旋操作。

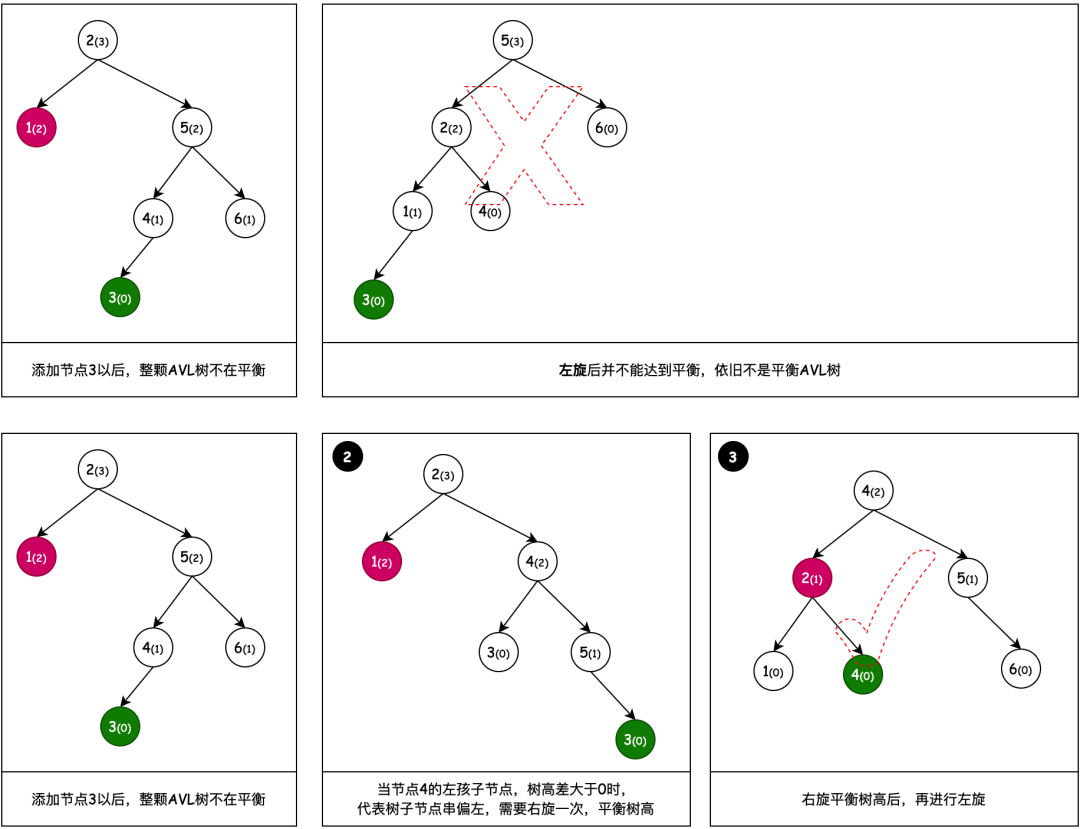
代码实现

if (factor(node.right) <= 0) {
    Node temp = super.rotateLeft(node);
    refreshHeight(temp.left);
    refreshHeight(temp);
} else {
    Node temp = super.rotateRight(node.right);
    refreshHeight(temp.right);
    refreshHeight(temp);
    node.right = temp;
    
    temp = super.rotateLeft(node);
    refreshHeight(temp.left);
    refreshHeight(temp);
}

四、AVL树功能测试

为了验证AVL树的实现正确与否，这里我们做一下随机节点的插入，如果它能一直保持平衡，那么它就是一颗可靠 AVL 平衡树。

单元测试

@Test
public void test_random() {
    AVLTree tree = new AVLTree();
    for (int i = 0; i < 30; i++) {
        tree.insert(new Random().nextInt(100));
    }
    System.out.println(tree);
}

测试结果

输入节点：61,3,34,82,1,75,56,65,87,18,3,96,53,50,42,24,69,11,95,69,1,1,84,22,5,70,28,55,38,92

                         /----- 96(0)
                 /----- 95(1)
                 |       \----- 92(0)
         /----- 87(2)
         |       |       /----- 84(0)
         |       \----- 82(1)
 /----- 75(3)
 |       |               /----- 70(0)
 |       |       /----- 69(1)
 |       \----- 69(2)
 |               \----- 65(0)
61(5)
 |               /----- 56(1)
 |               |       \----- 55(0)
 |       /----- 53(2)
 |       |       |       /----- 50(0)
 |       |       \----- 42(1)
 |       |               \----- 38(0)
 \----- 34(4)
         |                       /----- 28(0)
         |               /----- 24(1)
         |               |       \----- 22(0)
         |       /----- 18(2)
         |       |       \----- 11(1)
         |       |               \----- 5(0)
         \----- 3(3)
                 |       /----- 3(1)
                 |       |       \----- 1(0)
                 \----- 1(2)
                         \----- 1(0)


Process finished with exit code 0