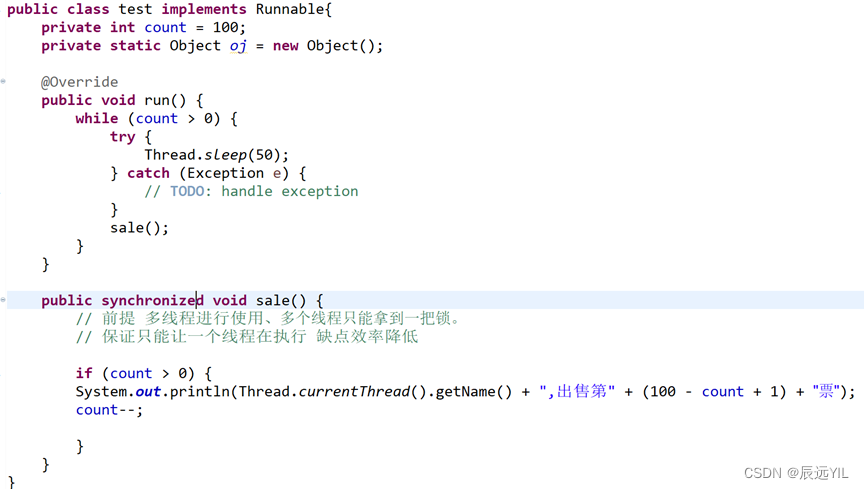
C++中的螺旋矩阵？
C++中的螺旋矩阵？
提问于 2018-01-31 09:27:26
我有一个程序，我必须用从1到n的螺旋形式来填充矩阵a。然而，我的程序只填充矩阵的边框。我的问题是如何优化算法，使其填充整个二维数组。
#include <iostream>
using namespace std;
void print();
const int n=9;
int a[n][n];
int main()
{
int counter=1,j=0,i=0;
for(int i=0; i<n; i++)
{
a[i][j]=counter;
counter++;
}
counter--;
for(int k=0; k<n; k++)
{
a[n-1][k]=counter;
counter++;
}
counter--;
for(int i=n-1; i>=0; i--)
{
a[i][n-1]=counter;
counter++;
}
counter--;
for(int j=n-1; j>0; j--)
{
a[i][j]=counter;
counter++;
}
print();
return 0;
}
void print ()
{
for(int i=0; i<n; i++)
{
for(int j=0; j<n; j++)
{
cout<<a[i][j]<<'\t';
}
cout<<endl;
}
}回答 1
Stack Overflow用户
回答已采纳
发布于 2018-01-31 09:57:55
有几件事需要在推特上发布：
- 你需要围绕你已经拥有的另一个循环来重复你每一轮螺旋的圆圈运动，并通过每次迭代减少圆圈的大小(在我的例子中，这是
m，它被简化为零)。 - 在主函数开始时初始化
int i和int j，但在循环中使用for (int i=0;...)，这意味着您没有使用以前定义的整数的值。
最后这看起来是这样的：
#include <iostream>
using namespace std;
void print();
const int n=6;
int a[n][n];
int main()
{
int counter = 1;
for (int m = n; m > 0; m--)
{
int j=n-m,i=0;
for(i=n-m; i<m; i++)
{
a[i][j]=counter;
counter++;
}
counter--;
for(int k=n-m; k<m; k++)
{
a[m-1][k]=counter;
counter++;
}
counter--;
for(i=m-1; i>=n-m; i--)
{
a[i][m-1]=counter;
counter++;
}
counter--;
for(j=m-1; j>n-m; j--)
{
a[n-m][j]=counter;
counter++;
}
}
print();
return 0;
}使用您的print()，这将产生以下结果：
1 20 19 18 17 16
2 21 32 31 30 15
3 22 33 36 29 14
4 23 34 35 28 13
5 24 25 26 27 12
6 7 8 9 10 11注意，我在这里选择了n=6。
页面原文内容由Stack Overflow提供。腾讯云小微IT领域专用引擎提供翻译支持
原文链接：
https://stackoverflow.com/questions/48548063
复制相关文章
相似问题
让printf线程安全吗？
方法是否从单独的线程调用，在调用线程上运行？
是否可以在c中同时运行主线程及其子线程？
在单个线程中调用和销毁类实例时线程是否安全？
同时从多个线程调用写()安全吗？
添加站长 进交流群
领取专属 10元无门槛券
AI混元助手 在线答疑
关注 腾讯云开发者公众号
洞察 腾讯核心技术
剖析业界实践案例
腾讯云开发者
